LIMITS

A good friend of mine, when he was learning to ride always wanted to know what the actual limits were.  Apparently I never answered him preferring to encourage him to learn the skills to feel for grip, measure his lean angle etc.  But it is a good question;

Throughout this article I will make reference to Steve Mundane’s website and efforts (http://www.stevemunden.com); whilst developing the maths I discovered Steve’s site and his work along the same lines.  Steve has approached it from a slightly different perspective and math but his work is fabulous and well worth the read.  Further he has given permission for it to be shared with you.

What exactly are the limits of a motorcycle

So seriously has anyone ever really answered that for you?  I mean not some wimpy idea about feeling for traction, like some of my articles, but an empirical discussion about the limits? What are the limiting factors???  Could Stephen Hawkins simply reduce this to a calculation??

During this short read I will attempt to take you on a journey, so bear with me.  The journey will explain the maths that affects a motorbike, prove the limits of cornering, braking and accelerating as a result, discuss what happens when the rules change (i.e. you overstep those limits) and then attempt to bring all this back around to usable information that in some cases will show you that you already reach the limits quite often and being smooth is what you should be trying for, whereas in others you’ll see that the limits are a safe distance away.

Be prepared to have some of your assumptions about motorcycling turned up side down!!!!

So keep reading to the end.. the really good stuff comes then, but only really makes sense when you understand why I can say the things I do!!!

Lets look at some maths. 

I often rant on about Newton’s Third law of motion, well his second law of motion is of a lot of interest here. 

Newtons Second Law States:

A body of mass m subject to a net force F undergoes an acceleration a that has the same direction as the force and a magnitude that is directly proportional to the force and inversely proportional to the mass, i.e., F = ma. Alternatively, the total force applied on a body is equal to the time derivative of linear momentum of the body.

Wow!!  that’s almost english.  But in the back of our minds we all know the old equation of Force = Mass * Acceleration (F=MA). 

So looking at corners first we can calculate the forces that are in effect.

One of the forces is of course the weight of the bike.  This force become quite important in our calculations.  The actual force is calculated by Weight * the acceleration of gravity, but we can simply.

Another force we need to consider is the speed we are travelling at.

The third force we are dealing with is the Centrifugal force or that force that your tires resist in order to keep you from just sliding off the road.  This force is made up on the tightness of the corner (which we will represent as a Radius in metres).

To calculate that I’m going to take these steps:

• Using the Radius of the turn calculate the circumference (using C= PI * 2 * R)
• Calculate the Revolutions per minute we would do around this circumference at our speed using (RPM = (((KPH*1000)/60)/Circumference ))
• Then we convert that to Radians per second using (Rad/sec = RPM/60*(2*PI()))
• Then we can calculate the Newton force based on (Weight * Radius * (Rad/sec*Rad/sec))
• Converting Newtons to KGf (Kilogram force) is simply a matter of Multiplying by 0.101971621

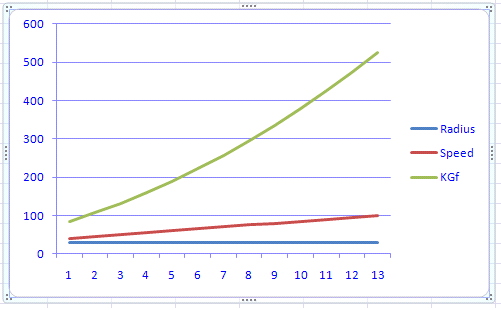
As you can see the centrifugal force rises much faster than your speed for a given corner Radius (This graph is for a 30m corner radius and a 200Kg Bike Rider combination)

so I guess the first limits we need to look at are HOW MUCH FORCE CAN A TIRE ACTUALLY HOLD??? and I guess as we are leaning to turn around corners HOW MUCH LEAN CAN A MOTORCYCLE SUSTAIN?

Well lets look at lean angle first

We can calculate lean angle if we know the forces involved.   The two forces involved are:

• Force pushing the bike out of the turn (Centrifugal force – which we just calculated)
• Force able to resist that (This is the weight of our bike and rider)

Then knowing these we can calculate the lean angle for a given speed and corner Radius because we know the old laws of force in balance (Newtons First Law of Motion)

First law: Every body remains in a state of constant velocity unless acted upon by an external unbalanced force.^[3][4][5] This means that in the absence of a non-zero net force, the center of mass of a body either remains at rest, or moves at a constant velocity.

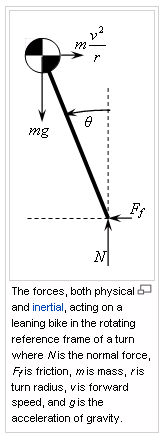
The formula is relatively simple, it just uses the information we already have and good ‘ol trigonometry to work out the lean needed to balance.    I’ll spare you the details but let you see the results. 

Interestingly enough we find that the weight of the bike/rider is actually irrelevant because it shows up on both sides of the equation i.e. the heavier bike produces more centrifugal force but leans the same to counter this as a lighter bike, so we actually end up with a constant that can map the G force of the turn against the lean angle required.  There is no way around this really.. the faster you go the more you must lean.  A small difference will come about due to Centre of Gravity changes between bikes , but it is pretty small.

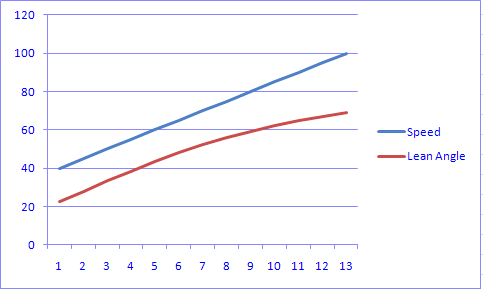
Ok.. so the faster we go round a given corner the more we lean, pretty easy to work out those limits.

A modern sports motorcycle may possibly have enough clearance to lean up to 57 degrees, most will not do better than 50 degrees.

So we can see above this will limit our speed in this 30m Radius corner straight away to less than 60Kph, but is that the first limit we will strike??  The next thing we have to look at is grip.  How much Kerb Thrust (Centrifugal force) can our tires hold onto?  Are heavier bikes going to be more limited than light ones?

To do this we need to look at a thing called FRICTION and the coefficient of Friction

Basically what we call grip on our tires, scientists call the coefficient of Friction or µ (Moo).

But before we get into that perhaps we should explain the laws of friction.  Firstly there are three main types of friction:

• Dry Static Friction
• Dry Kinetic Friction
• Lubricated Friction

The first of these is what we really need to concentrate on as this is the grip we get on a dry tarmac when we haven’t already started sliding.  Dry Kintetic friction is what we start to work with once things let go.

Now Dry Static Friction has a number of laws some of which are going to rott your socks.

• Amontons’ First Law: The force of friction is directly proportional to the applied load.
• Amontons’ Second Law: The force of friction is independent of the apparent area of contact.
• Coulomb’s Law of Friction: Kinetic friction is independent of the sliding velocity.

The first law makes sense right?  I covered this off in the article “RoadCraft – Finding Traction”.  The more weight you use to press two surfaces together the more force you need to get them to slide. 

This is actually where the µ comes in, it is actually the factor that decribes for two specific materials what the ratio between the force pressing them together and the force needed to slide them is.  So if µ was 0.7 for rubber on tarmac and you had 100Kg pressing the rubber to the tarmac it would take 70Kg of sideways force to slide that rubber.

The second law is the one that gets everyones goat!!!  The force of friction is NOT dependent on the surface area of the surfaces in contact but ONLY on their coefficients of friction and force pressing them together.

I Know you will immediately argue “what about bigger tires.. they must give me more traction???”.  The answer is explained in “RoadCraft – Finding traction” but for simplicity think about this mental exercise.

You hold in your left hand a tiny 110 tire sitting on the tarmac, in your right hand  you hold a might 190 tire also sitting on the tarmac.  Which one takes the most effort to drag across the road toward you??  How will that change if I tell you the 190 is just a tire by itself and the 110 has a 200Kg bike pressing down on it?  I’ll leave you to struggle with that for a moment but the truth is your not lifting the 200Kg just dragging it.. the frictional effort has obeyed the first and second laws.

The third law just says, in motorcyclist terms, when you start sliding the game changes and quite dramatically for us!!!

So what is the µ of Rubber on Tarmac?

Well it varies? depending on the rubber, tarmac and yes heat of the rubber. 

Rubber is one of the few materials who’s µ changes as it heats.  This is because of a thing call molecular reformation, i.e. rubber changes molecular structure as it gets hotter.  One issue here is that as it gets hotter it will eventually reduce the grip dramatically as it effectively starts to Melt

Ok, so that is not the answer you wanted was it!!  So here’s a better one and more importantly a pretty accurate one because we are most interested in the absolute limits. 

The number varies between 0.7-1.25 for a normal road tire on dry tarmac, dependent on heat and softness of tire to begin, with under normal conditions.

Ok that we can work with a theoretical maximum of  approximately 1.25 for a sports tire on dry tarmac.

So for say a 200Kg bike and rider we can work out how much Kerb thrust (centrifugal force) is generated for a given corner.  We now know that the maximum kerb thrust that a modern sports tire will hold with 200Kg pushing it onto the tarmac would be 200Kg * 1.25 or 250KGf.

We also know we can work out the lean angle that would be needed given a corner kerb thrust of 250Kg, which if you not as quick as my spreadsheet is 51 degrees of lean.

SO THE FIRST REAL LIMIT WE CAN FIND IS NOT THE LEAN ANGLE LIMIT OF A GOOD MODERN SPORTS BIKE, BUT THE GRIP OF A GOOD MODERN ROAD SPORTS TIRE.  WE ALSO KNOW THAT IF WE ACHIEVE CLOSE TO 50 DEGREES OF LEAN THEN WE HAVE ACHIEVED CLOSE THE TO THE MAXIMUM CORNER SPEED (at least for part of the corner)

We have also learnt the next valuable limit

How far can you lean a bike? Until you run out of ground clearance or approximately 50 degrees (On high performance road tires)The graph below shows the maximum corner speed for a given radius of turn (of course all at the maximum lean of approx 50 Degrees and for a maximum 1.25µ)

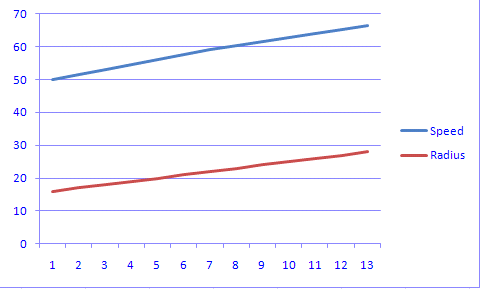
Have you noticed that µ (the coefficient of friction) maps very nicely onto the G force (magnitude of Gravity), Maximum Kerb thrust isequal to the same force that Gf * Mass would be if Gf = µ.  If this is true and the maximum µ of a tire really is approximately 1.25 then if we were to do emergency braking exercises the resulting minimum stopping distances that we could predict using maximum Gf (G force) would be when the Gf = 1.25.

Well it appears there is lots of data for motorcycle emergency braking and if you take all this data and feed it into Newtons second law (F=MA) you can calculate the acceleration (or Gf) that was achieved and guess what… it in the range of 1.1-1.2 (for sports tires and a little less for harder tires).

Which also means:

The limits of stopping distance on a  modern motorcycle with good brakes are the limits of traction of your tires (in most cases, although lighter bikes could be limited by keeping the rear wheel on the groundSo of course if you can increase your µ you can get higher corner speeds and more lean, just look at our MotoGP hero’s that are riding on soft as toffee slicks and getting over 60 degrees of lean at times.  

So how much difference does it make if you are on lesser tyres?

Well maximum lean angles, and therefore maximum µ, for less sporty tires are not usually quoted, however we know from experimental testing that other factors excluded, the minimum breaking distance achievable for a given tire maps pretty accurately onto the tires µ.  (Given brakes that are powerful enough etc).

Those we know and it would appear that even a relatively hard compound tire is able to reach a µ of 0.8 under dry condition, which is roughly the same as a tire capable of a µ of 1.25 (Dry) in the wet, for which we do know the lean angles.

So how much difference does it make?  The difference between a soft sports compound and a harder touring tire (representative of a 0.8 – 1.25 µ gap) would be approximately 10Kph of corner speed or 10 degrees of lean angle.

So the difference a tire makes is huge!!  In fact in theory you can ride a soft compound sports tire as hard in the wet as you could a touring compound in the dry.

So what are the hard limits of a motorcycle?

in absolute terms we have discovered the limits are:

Maximum lean angle that your bike is capable of which will be in the range of 45-57 degrees

Maximum µ that your tire can achieve, which for a sports road tire on a hot day with good road surface will see not much more than 1.25 or a lean limit of 50 degrees.

But a word of caution!!!

In real terms your bike limits are directly related to your tires maximum grip which in turn relates to your tires µ or coefficient of friction.  Under perfect conditions with a good quality sports tire this can be as high as 1.25 and for most tires on the market will at least reach 0.8.  But what happens when you take into consideration changing road surfaces or other things that can affect the friction coefficient???

Typical static Coeff of Friction values	Low	High	Wet
Concrete		0.55	1.2	-20%
Asphalt		0.5	0.9	-20%
Gravel		0.4	0.8	-20%
Ice		0.1	0.25	-20%
Snow		0.1	0.55	-20%

As you can see from the table above the grip offered by your tires can vary alot!!  and it gets worse, because so far we have been talking about the grip that is offered by Static friction coefficient not by the kinetic friction coefficient (which is in the table below).

Asphalt (Wet)	0.25-0.0.75
Concrete (Dry)	0.6-0.85
Concrete (Wet)	0.45-0.75
Asphalt (Dry)	0.5-0.8

So once you start to slide your grip actually reduces (explains why that locked wheel won’t stop you under brakes).  I’ll write another time about the dynamics of a slide.

Your maximum limits are determined by your maximum grip and on the road that can change dramatically and suddenly!!! be warned.

But trust me on this absolute, unchanging certainty

ALL THINGS BEING EQUAL YOUR LIMITS ARE DICTATED BY YOUR TIRE CHOICE.